I’ve been curious, how does one measure the tomography? There are polarizers in front of the detectors, and we’ll be measuring correlations of counts from the two detectors. If one is found to be H then the other one ‘collapses’ H. But this could be the mixed state, and not entangled. Where is the interference? If you have time, thanks for straightening out my misunderstanding.
Judges’ Queries and Presenter’s Replies
Presentation Discussion
-
-
Your understanding of the tomography setup is correct if we only used polarizers like you describe. If one is found to have H polarization, the other could collapse to H (state dependent), but this does not prove that the state is entangled at all.
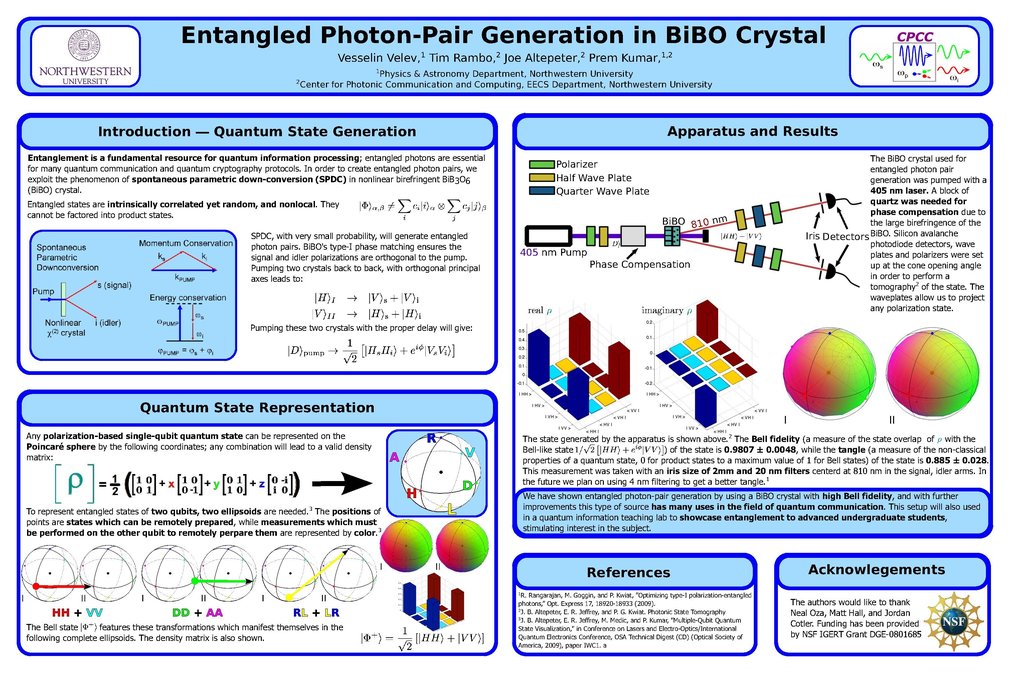
Just like a CT scan takes slices of the human body from different orientations to piece together a 3D reconstruction of the original, we do the same thing with the grouping of half-wave plate, quarter-wave plate, and polarizer for each entangled pair before each detector which can be seen on the poster under the ‘Apparatus and Results’ heading. This grouping of optical elements allows us to project onto any point on the Poincare sphere (the different orientations for our reconstruction). We measure in all 3 orthogonal bases (H-V, D-A, L-R), which are represented by each of the axes in the sphere. Recording the coincidences in the two detectors while measuring all 36 bases combinations for the pair of detectors allows us to characterize the two-qubit state that was generated.
In our measurements, we always set the polarizer to H, and rotate the wave plates in order to project H,V,D,A,L, or R onto the detector. We start out by setting the grouping of waveplates for the top detector to project H, and for the bottom to project H. Then we keep the top at H, and set the bottom to V. Then top to V, bottom to H, then both to V. This is a measurement in the H-V basis for qubit 1 and H-V for qubit 2. We do all combinations of each basis for qubit 1 with each basis for qubit 2, hence 36 measurements. While this set is over-complete, in the sense we are finding 36 parameters for 16 quantities (each element of the 4×4 density matrix), it is more robust to variations in pump intensity and differences in detector efficiency. Once this set of 36 measurements is complete, a maximum likelihood technique is used to find the legitimate state most likely to have generated those measurements. The results of this density matrix are then visualized using two spheres as shown on the poster.
Feel free to ask any follow-up questions!
-
Further posting is closed as the event has ended.
Judges and Presenters may log in to read queries and replies.