I received a question, so I am posting my reply here in case other people have the same question.
- - - - - - - -
Hi Tristan,
this is fascinating work. What are its implications? Where do you think this will be most important?
Thanks
- - - - - - - -
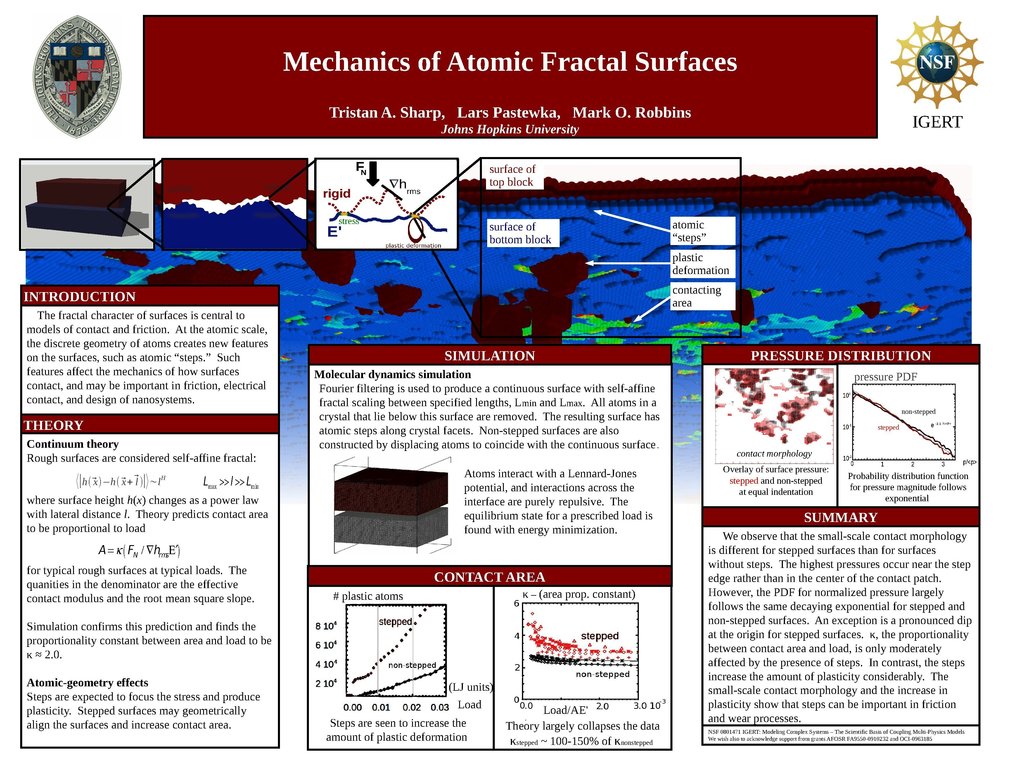
Thanks! We think so too. If we understand how the surface geometry of solids can change contact properties, then we’ll be closer to understanding friction, wear, adhesion and several other fundamental processes that engineers mostly treat phenomenologically right now. What’s more, this all becomes critical when designing nano-scale machines, where these processes dominate. These results presented here are a piece of the puzzle. Hopefully this work will contribute to the dialog as we try to discover what’s important in engineering at the atomic-scale. Atomic-scale engineering, of course, promises great advances in medicine, energy, communications, etc.

Another way to put the results succinctly is the following: We see evidence that there is little need to incorporate “steps” into the theory of rough contact if the only quantity of interest is contact area. (A researcher trying to interpret thermal conductivity data may wonder if the sample’s stepped surface affects the amount of area touching.) However, the steps produce stresses that are not predicted in step-less theories, and so therefore steps may be necessary in a more accurate theory of wear, for example. (An engineer designing a submicron mechanical device may wonder if a material without surface steps is necessary to diminish wear.)

Hope this answers your question.

Hi Tristan. Fascinating research… What kind of computational power do you need to run these calculations: desktop computing? HPC computing? Supercomputing? If I understand correctly you are using repulsive LJ interactions in your simulations: what are the limits of this approach: why not calculating attractive interactions as well, and including the dynamics of the materials? Could this lead to some kind of “relaxation” or diffusion of the stress?
- - - - - - —
We need to simulate large systems to escape finite-size effects and get enough asperities (peaks) to contact. Our IGERT participates in IDIES, the Institute for Data Intensive Engineering and Science at Johns Hopkins University. This gives us access to an HPC cluster and GPU cluster where I run these simulations on hundreds of processors.

We use full LJ interactions in the solids and repulsive LJ interactions between the solids. We use LJ and don’t attempt to perfectly model any one specific material, because we are trying to ask general, more widely-applicable questions.

Adhesion, since it is often present, is actually in our plans to add as we continue this work! Similarly, dynamics would be great to investigate, though typical dynamical loading would relax to these static solutions. There wouldn’t be any difference in the stress.

Thanks for the question.

Another question: what code are you using, did you develop it yourself?
- - - -
We use the parallel code LAMMPS, introduced by S. Plimpton J Comp Phys, 117, 1-19 (1995). The code was extended with a multi-scale method, introduced by Kong, Bartels, Campana, Denniston, and Muser Comp Phys Comm, 180, 6 (2009). Then further adapted by us.

Hi Tristan,

You’ve did phenomenal work on your online poster. I look forward to your explanation in laymen’s terms at the annual IGERT meeting.

Why is plasticity dependent on the steps?
- - - -
The step edges seem to concentrate the stress, just like the rigid punch solution in linear elasticity. We see a corresponding increase in the plasticity, as we try to show in a plot at the bottom of the poster. There we plot the number of atoms that have left their FCC environment.

What would be a real world example of stepped surface interactions versus non-stepped interactions? Would it be safe to say that two different objects that have completely different chemistry would more often act as a non-stepped interaction?
- - - -
Stepped surfaces are very common on many metals, for example. Atoms that tend to form a lattice naturally produce these steps at the surface, because it is energetically favorable. The steps can be viewed in STM images and are simply due to the positions of atoms.

Amorphous materials like glass that do not form a lattice do not display these stepped surfaces. Also, bending a lattice can produce an atomically-smooth (non-stepped) surface.

Another question is about chemical interactions at the surface. In the work presented here, we have simply used repulsive Lennard-Jones potentials between the objects. We wanted to isolate the effects of step geometry without introducing additional chemistry. I hope this answers your question!

Can you explain the right graph in the pressure distribution panel?
- - - -
The right-most plot is the probability distribution of pressures on the surface. It is the probability to find an atom carrying a certain force. Looking at the middle of the plot, we can read off that the probability that a contacted atom carries twice the force as the average contacted atom is about 10%. The steps don’t appear to change this. (Possibly plasticity relieves high stress at the step edges.) If there were a long tail, for example, that would mean many atoms carry loads much higher than the mean load in the contact.